I just read a really interesting post called ‘Applying Variation Theory.’ It’s by a teacher from the UK who I don’t yet know, Naveen Rizvi. The core mathematics is familiar territory to teachers of algebra. To factor an expression like , you can ask yourself “what pair of numbers sum to 10 and multiply to 16?”
Answer: 8 and 2.
Therefore:
I actually first learned about this from Dan Meyer, and for my first few years teaching quadratic factoring I used his “Diamond Problems” as a factoring lead-in:

In her post, Naveen talks about intentionally designing problems of this sort to draw out this underlying structure — that the factors of “c” have to sum to “b.” Factoring then becomes a quest to search the factors of “c” for things that sum to “b” (or vice versa).
Her main points is a good one, which is that if you keep everything else the same, and then vary just one thing, that thing will draw a lot of attention. Here is how she uses these ideas to design a practice set of quadratics, one that isn’t so unlike Dan’s:
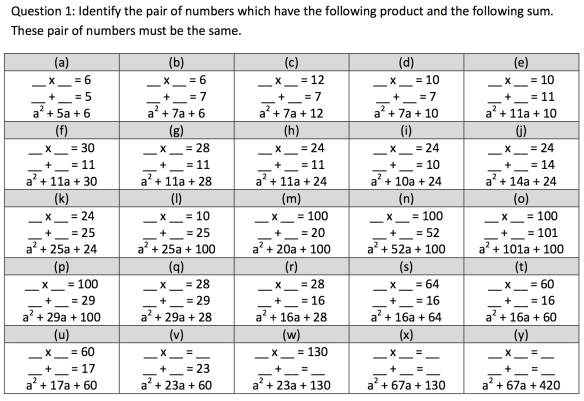
My experience of teaching this topic is that, even knowing the relationship between c and b, these problems can be very difficult for students.
Why? What makes these factoring problems hard? Part of the reason has to do with fact automaticity, to be sure. While there are many topics in algebra that a student can handle without automaticity, this is definitely one of the times when things get much hairier for kids if they don’t know e.g. quick ways to find all the ways of multiplying to 60.
But take a closer look at some of these problems, and you can see that there is more going on than just knowing the relationship between b and c and knowing your facts. Consider one of these problems from Naveen’s page, which I just chose randomly:
This is a problem that I can imagine my students having some trouble with, at first. Not because the facts are difficult or because they don’t know the relationship…it’s just that the solution (a + 1)(a + 28) might not occur to kids. One thing I’ve noticed is that a lot of kids don’t think of 1 x N for a while when they’re searching for ways to make N. This makes sense — it’s so computationally straightforward, they don’t spend a lot of time thinking about multiplying by 1. It can slip under the radar.
Now, might a kid working on Naveen’s problem set become familiar with this tiny nugget or structure, that if the “b” term is one off from the “c” term, you should try sticking “1” into one of your binomials?
A student might make this generalization from the examples in the practice set, but this would essentially amount to learning by discovery. Which absolutely happens sometimes, but especially since this activity isn’t structured around giving kids instances that would cue-up that generalization…it probably won’t happen for most kids.
Now, the question is whether this sort of “micro-strategy” is a good use of classroom time. Maybe it’s too narrow a class of problems to be worth making it an instructional focus, I don’t know. Maybe you just go for the main strategy, and hope that kids are able to apply what they know to this little side-case.
But then again, maybe you give a quiz and kids end up mostly baffled by this problem. Teaching is full of surprises — this could happen.
That’s when I say, OK, let’s design a quick activity that would focus entirely on this micro-skill. Maybe a mini-worked example, or maybe a string of mental math factoring problems ala Naveen or Dan’s that puts that entire “variation theory” focus on this one, specific corner of the mathematical landscape — just the one that the students in your class need.
And then I call this “feedback” and don’t spend a lot of time writing up stuff in the margins of their quizzes.
There’s something nice about these little micro-skills. For one, it’s an alternate way of thinking about what’s leftover after you’ve taught the “main” skill. (Meaning, it’s not just that kids are forgetting what you’ve taught and need to be reminded — it’s that there are little corners of the mathematical world that haven’t yet been uncovered for kids.)
One thing I’ve been struggling with has been trying to figure out what exactly my pedagogy involves that’s distinctive. It’s not about the activities I tend to choose or design — since I’m pretty boring in that regard. I’m not an amazing motivator of people. Kids like me, I think, but not in the “oh my god he was my best teacher” way. More like “he’s nice.” (“Being nice” is an important part of my pedagogy.)
Though I still don’t have a snappy way to put it, I think that this is part of my story:
- I’m really curious about how kids think
- So I try to use that to come up with a more systematic understanding of how they think about different types of problems, especially when it’s something that people typically think of as constituting a single “type” of problems (e.g. factoring quadratics, solving equations, adding fractions)
- While teaching a topic I try to figure out which types of problems the kids understand how to handle, and which they don’t yet
- Then I focus in on a micro-skill for handling one of those little types of problem and I teach it with a short little activity followed-up by practice, in place of feedback
I don’t think any of this is exciting or inspiring, and I don’t really think I can make it so. There really is something here, though.
I think the exciting action comes in the second of those bullet points, in describing the mathematical landscape in a way that’s pedagogically useful. One of my favorite things in math education is Carpenter et al’s Cognitively Guided Instruction. I’ve moved away from some of the pedagogy it has grown into, but the problem breakdown is my paragon of pedagogically useful knowledge. It’s what I always come back to.
I sometimes wish there was more to what I do, and I also wish I didn’t have such a hard time figuring out how to describe what it is that I’m into. I’ll be doing a thing with teachers this spring that will give me another shot at refining my little message. I sometimes get jealous when I see all the cool things that people make and share online. I’ve never been cool, and none of what I’ve written here is cool either, but it’s what I’ve got.
(Last year’s version of this post: here.)