What should count as an explanation in math? Even though most people in education think that explanations are important, they often struggle to clearly define what an explanation is. This has led to a situation where it’s relatively easy to make fun of mathematical explanations: You want kids to explain why 2 + 3 is 5? Solve the problem, what other explanation do you need!
In support of the pro-explanation camp, there is a large body of evidence that finds that it helps people when you prompt them to explain things to themselves during their learning. And this indeed does seem to settle the case, until we ask the exact same question as before: what does it mean to explain something to yourself?
Making it even more confusing? The use of “self-explanation” by researchers is a moving target. Sometimes it is supposed to be self-talk, a coherent explanation to yourself; other times it’s just any old inference you’ve made, even if it doesn’t add up to an “explanation.” Sometimes it counts as self-explanation if you say it aloud. Other times self-explanation is supposed to be an exclusively internal phenomenon.
I indeed found this extremely confusing, but felt much clearer after I read a fantastic piece by Alexander Renkl and Alexander Eitel that lays the whole situation out in a coherent way. First, they note that the situation is indeed very confusing and the meaning of “self-explanation” has changed over time:
As use of this construct has become so widespread, its meaning has changed. For example, Chi (2000) now sees (at least some) self-explanations as the process by which learners revise and improve their yet imperfect mental models. Presumably due to the widely varying use of this construct, its recent characterizations are rather general such as “inferences by the learner that go beyond the given information” (Rittle-Johnson et al., 2017) or explaining “the content of a lesson to themselves by elaborating upon the instructional material presented” (Fiorella & Mayer, 2015, p. 125). If one relies on such definitions, it is hard to draw the boundaries between other established constructs in research on learning such as “elaborative inferences” (in text learning; Singer, 1994) or “elaboration strategies” (Weinstein & Mayer, 1986). It is also questionable whether all the phenomena labeled self-explanations in previous research can be justifiably called explanation (for discussions about the concept of explanation, see, e.g., Keil, 2006; Kiel, 1999; Lombrozo, 2012).
Then they say something very sensible, which is that self-explanation is probably not one but rather many phenomena, related but distinct:
Given that even the initial characterization of self-explanation already included relatively heterogeneous phenomena (see the four findings reported by Van Lehn et al., 1992) and given that the subsequent extensions led to very general characterizations, there would appear to be little justification for discussing the learning effects of self-explanations and their use in (classroom) instruction on a general level. It is highly probable that different types of self-explanations have different functions, lead to better learning via different mechanisms, and should not be regarded as a unitary construct when providing practice recommendations.
They then announce that they are going to focus in on just one particular form of self-explanation. They call it “principle-based self-explanations” but that isn’t terribly evocative. What they’re describing is connecting the particular details of whatever it is we’re looking at to generally applicable patterns or rules. This form of self-explanation is a form of explanation; it’s explanation by way of connecting these specifics to that general rule:
We focus on principle-based self-explanations (Renkl, 1997): Learners “self-explain” a step in a problem solution (e.g., physics problem) or a feature of an object (e.g., the appearance of an animal) in reference to an underlying principle (e.g., one of Newton’s laws or mimicry). Such self-explanations were part of the initial self-explanation concept (e.g., VanLehn et al., 1992). They can also reasonably be called explanations. Although the concept of explanation can have different meanings (e.g., Keil, 2006; Lombrozo, 2012), explaining a case in terms of underlying principles is a quite prototypical case of explanation. This type of explanation fits the subsumption and unification accounts of explanation (e.g., Lombrozo, 2012). These accounts regard a case as explained when it is subsumed under a more general pattern (e.g., Newton’s laws or the general strategy of mimicry).
Let’s come back to 2 + 3 = 5. How could you connect this to some general principles? It wouldn’t just be taking two things and three more things and counting them. That’s of course valuable, but to get the benefits of what Renkl and Eitel are talking about it would have to be something more abstract and general. It probably shouldn’t involve the words “2” or “3,” or at least that’s not the important part. Maybe you’d say, “Whenever you are adding you can count on from one number by the other number.” Or maybe “You can always put both numbers on your fingers and count them together.” The point is the always. You are seeking to connect the particulars of this to a general pattern that repeats a lot.
The benefits of this kind of self-explanation, Renkl and Eitel write, are to be better prepared to apply that generalization to future cases. This makes sense since you are articulating a generalization, in the process abstracting some example or solution and turning it into a generally useful principle, procedure, strategy, structure.
They even have a nifty diagram:

I think this is the simplest way to think about what a mathematical explanation is, or what it should be aiming for. It’s simply asking students to make a generalization. Articulating a generalization, if one is able to, is pretty clearly going to help students handle future cases that fall under that general pattern.
Just as equally, if students aren’t yet able to articulate a generalization then they probably won’t get much out of being asked to explain. A lot of the cases that are easiest to make fun of are situations where students just don’t yet know how to articulate those generalizations. If students don’t know how to explain 2 + 3 = 5, they can be taught what those general principles are explicitly and then asked to apply that to other problems such as 4 + 5 = 9 or whatever.
One of my favorite techniques I’ve been playing with this year is giving students two generally applicable principles and asking which better explains a given solution. This removes the burden of articulation from the kids, and instead asks them to focus on deciding which abstraction best matches the particulars. And if it’s sometimes ambiguous (as arguably the choices below are) that can be OK too, since it’s all still about connecting particulars to general principles.
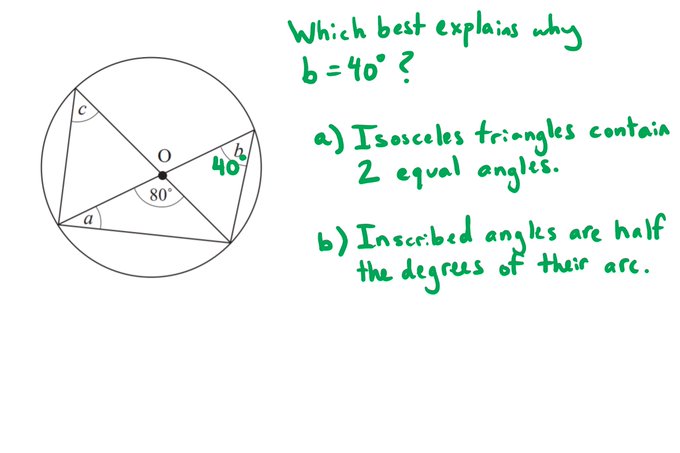
Practically, this means that teachers can be clearer to students about what we’re looking for. We can ask students to tell us something that is always true, or that will always work. We can teach that great explanations aren’t just about this problem, but that they show how a particular instance is part of a larger pattern. In this way we can teach students how to give good explanations, at first to others. But my guess is with time this habit is internalized, and you start giving better explanations to yourself.
Hi Michael,
Nice post!
Chi (2000) offers an additional mechanism:
(1) Our mental models are faulty.
(2) A prompt makes a conflict salient.
(3) As a result of that we self-explain.
(4) The self-explanation repairs the mental model.
(5) The end result is learning.
Better students see more conflicts, that’s why they self-explain more.
Do you see that causal mechanism as plausible?
Cheers,
Mario
I love Chi’s work! But doesn’t (4) leave you wanting to know what self-explanation is? Chi sometimes isn’t as clear as she could be about what self-explanation itself is. How does it repair the mental model?
In particular, is self-explanation something that passively happens once someone notices faults with their current models? Or is it some other active processing? And if so, what is that processing? That’s where I think this post comes in.
Thanks Michael, that makes a lot of sense.
Faulty mental model ➔ conflict ➔ self-explanation ➔ connecting problem cases with principles ➔ self-repair ➔ learning.